| Chapter
16 Handout on Rarefaction Calculation
|
BIOL
4120
Principles
of Ecology |
 |
 |
 |
 |
 |
 |
|
Harned Hall 301
(615) 963 - 5782 |
| |
| Above: Eucalypt trees from Australia growing in Brazil
to provide fiber for disposable diapers. The newly planted trees are
in the foreground and the dark green band behind them is the forest
after only 5 years! The native ecosystem, the Atlantic Forest, is
one of the most diverse and threatened terrestrial ecosystems.
|
The process of
rarefaction is necessary in order to compare species number (S)
from samples of different sizes.
Remember that
# of Fish from three lakes |
| Species
of fish |
North America |
Central America |
Argentina |
|
A |
12 |
|
|
B |
5 |
|
|
C |
4 |
33 |
|
D |
3 |
32 |
|
E |
1 |
34 |
|
F |
|
33 |
|
G |
|
|
42 |
H |
|
|
23 |
I |
|
|
16 |
J |
|
|
14 |
K |
|
|
6 |
L |
|
|
5 |
| |
|
|
|
| total |
25 |
132 |
106 |
=N |
| each cell is an Ni |
| # of species
|
5 |
4 |
6 |
=S |
To compare all
three lakes, we need to rarefy the samples from Central America
and Argentina to the smallest sample, North America
The book does not say,
but n must be THE SMALLEST SAMPLE SIZE
The
criterion is that N > n, or you will not be able to do the
combinatorials when N < n
Therefore,
rarefaction always adjusts down, never up.
So, we can only ask
"How may species would I have gotten in this sample if it
had been as small as the smallest sample?
We will use n = 25
from the North American A sample and rarefy the North American B
and Argentine samples
Central America |
| |
N |
n |
Ni |
N-Ni |
N-Ni n
Factorial |
N n Factorial |
fraction |
1--fraction |
C |
132 |
25 |
33 |
99 |
1.82E+23 |
6E+26 |
0.0003 |
1 |
D |
132 |
25 |
32 |
100 |
2.43E+23 |
6E+26 |
0.0004 |
1 |
E |
132 |
25 |
34 |
98 |
1.36E+23 |
6E+26 |
0.0002 |
1 |
F |
132 |
25 |
33 |
99 |
1.82E+23 |
6E+26 |
0.0003 |
1 |
| |
|
|
Total = |
4 species |
In the Central
American lake sample, we do not get much of a correction (too
small to show up). Why?
- This sample is
very even, and if you reduce the sample size, all four
species should be sampled, as all are about equally
likely to be sampled
This situation is a
bit different for the Argentine sample, where the sample is not
so even, although the richness is greater.
Argentina |
| |
N |
n |
Ni |
N-Ni |
N-Ni n
Factorial |
N n Factorial |
fraction |
1--fraction |
G |
106 |
25 |
42 |
64 |
4.01E+17 |
1E+24 |
3E-07 |
1 |
H |
106 |
25 |
23 |
83 |
1.08E+21 |
1E+24 |
0.0008 |
1 |
I |
106 |
25 |
16 |
90 |
1.16E+22 |
1E+24 |
0.0091 |
0.99 |
J |
106 |
25 |
14 |
92 |
2.2E+22 |
1E+24 |
0.0172 |
0.98 |
K |
106 |
25 |
6 |
100 |
2.43E+23 |
1E+24 |
0.1902 |
0.81 |
L |
106 |
25 |
5 |
101 |
3.22E+23 |
1E+24 |
0.2528 |
0.75 |
| |
|
|
| Total = |
5.53 species |
Here, there is a
noticeable correction. Why?
- The less
common species are much more rare than are the most
common, and so, they might not be sampled at all in a
smaller sample.
The last example rearranges the
Argentine data, but keeps the number of species (6) and total sample size the
same (106). What it does is make species G more dominant at the expense of all
other species (look at the Ni column here and compare with the previous Argentina
table).
| Argentina
- with almost all fish from species G |
| |
N |
n |
Ni |
N-Ni |
N-Ni n
Factorial |
N n Factorial |
fraction |
1 - fraction |
G |
106 |
25 |
80 |
26 |
26 |
1E+24 |
0.00 |
1.00 |
H |
106 |
25 |
9 |
97 |
1.01E+23 |
1E+24 |
0.08 |
0.92 |
I |
106 |
25 |
7 |
99 |
1.82E+23 |
1E+24 |
0.14 |
0.86 |
J |
106 |
25 |
5 |
101 |
3.22E+23 |
1E+24 |
0.25 |
0.75 |
K |
106 |
25 |
3 |
103 |
5.64E+23 |
1E+24 |
0.44 |
0.42 |
L |
106 |
25 |
2 |
104 |
7.42E+23 |
1E+24 |
0.58 |
0.24 |
| |
|
|
Total = |
4.50 species |
I included this
example for two reasons
- Reason 1 -
notice that the effect here is much more drastic because
the community is much less even. Now, you expect to get
only 4.18 species when you sample only 25 individuals.
- Reason 2 - Suppose that the number
of species G was 82 (and there were two less of Species K, to keep the total
the same). When you calculate this rarefaction, you run into an impossible
situation. The combinatorial in the numerator for species G is impossible
to calculate. It is 24 over 25. You can't calculate this, because it becomes
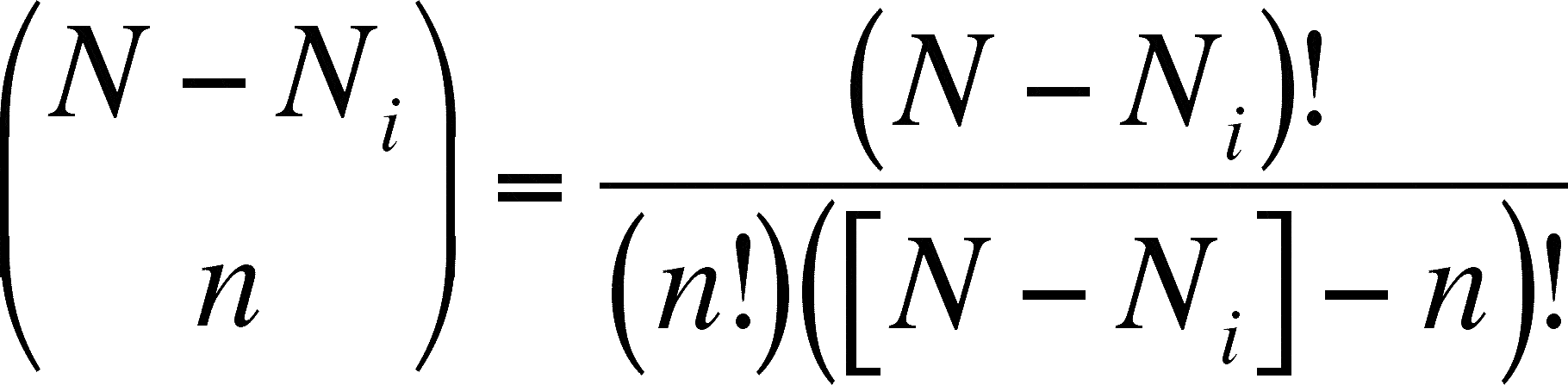
The -1 from the ((N - Ni) - n)
factorial (24 - 25) is the problem. I might just set it to 0 to do the calculations
in the table because
there is no such thing
as a factorial of a negative number and any combinatorial with this is 0
by definition.
So, we see that rarefaction
is a bit more involved than the text makes out, but still a worthy exercise
for the mind.
In
addition, honesty makes me disclose that this is not the only way
to rarefy a sample
For
example, one might use a bootstrap approach
(made possible by the speed of computers)
In
this approach, one subsamples a large sample repeatedly
and then calculates the parameter of interest based on
the subsamples
- For the above example on the
Argentine lake, one would make a subsample by
taking the 106 individuals in the sample and
randomly choosing only 25 of the 106 to be in the
subsample. One would then count the number of
species in the subsample and that would be one
bootstrap estimate for species richness
Next,
one would resample the original 106 individuals again,
choosing another 25 at random (some of those in the
second subsample could have been in the first) and
recalculate the number of species
Repeat
the subsampling many times (this is why a computer is
necessary, 10,000 is a good number of subsamples), each
time getting an estimate of the richness
Finally,
average the 10,000 richness values for the 10,000
subsamples and use that average as your rarefied estimate
of richness [ = E(S) in the formula above].
Last Updated on April 10, 2006